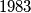
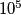
Is it possible to choose distinct positive integers, all less than or equal to , no three of which are consecutive terms of an arithmetic progression?
%V0
Is it possible to choose $1983$ distinct positive integers, all less than or equal to $10^5$, no three of which are consecutive terms of an arithmetic progression?
Izvor: Međunarodna matematička olimpijada, shortlist 1983
Školjka 
distinct positive integers, all less than or equal to 
, no three of which are consecutive terms of an arithmetic progression?