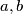
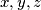
Let and be positive integers, no two of which have a common divisor greater than . Show that is the largest integer which cannot be expressed in the form , where are non-negative integers.
%V0
Let $a,b$ and $c$ be positive integers, no two of which have a common divisor greater than $1$. Show that $2abc-ab-bc-ca$ is the largest integer which cannot be expressed in the form $xbc+yca+zab$, where $x,y,z$ are non-negative integers.
Izvor: Međunarodna matematička olimpijada, shortlist 1983
Školjka 
and 
be positive integers, no two of which have a common divisor greater than 
. Show that 
is the largest integer which cannot be expressed in the form 
, where 
are non-negative integers.