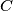
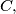
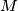
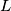
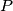
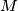
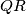
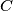
In the plane let be a circle, a line tangent to the circle and a point on . Find the locus of all points with the following property: there exists two points on such that is the midpoint of and is the inscribed circle of triangle .
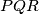
%V0
In the plane let $\,C\,$ be a circle, $\,L\,$ a line tangent to the circle $\,C,\,$ and $\,M\,$ a point on $\,L$. Find the locus of all points $\,P\,$ with the following property: there exists two points $\,Q,R\,$ on $\,L\,$ such that $\,M\,$ is the midpoint of $\,QR\,$ and $\,C\,$ is the inscribed circle of triangle $\,PQR$.
Izvor: Međunarodna matematička olimpijada, shortlist 1992
Školjka 
be a circle, 
a line tangent to the circle 
and 
a point on 
. Find the locus of all points 
with the following property: there exists two points 
on 
and 
.