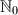
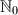
Let denote the set of nonnegative integers. Find all functions from to itself such that
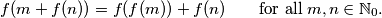
%V0
Let $\mathbb{N}_0$ denote the set of nonnegative integers. Find all functions $f$ from $\mathbb{N}_0$ to itself such that
$$f(m + f(n)) = f(f(m)) + f(n)\qquad \text{for all} \; m, n \in \mathbb{N}_0.$$
Izvor: Međunarodna matematička olimpijada, shortlist 1996
Školjka 
denote the set of nonnegative integers. Find all functions 
from 