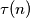
For any positive integer , let denote the number of its positive divisors (including 1 and itself). Determine all positive integers for which there exists a positive integer such that .
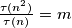
%V0
For any positive integer $n$, let $\tau (n)$ denote the number of its positive divisors (including 1 and itself). Determine all positive integers $m$ for which there exists a positive integer $n$ such that $\frac{\tau (n^{2})}{\tau (n)}=m$.
Izvor: Međunarodna matematička olimpijada, shortlist 1998
Školjka 
, let 
denote the number of its positive divisors (including 1 and itself). Determine all positive integers 
for which there exists a positive integer 
.