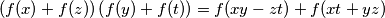
Find all functions from the reals to the reals such that for all real .
%V0
Find all functions $f$ from the reals to the reals such that $$\left(f(x)+f(z)\right)\left(f(y)+f(t)\right)=f(xy-zt)+f(xt+yz)$$ for all real $x,y,z,t$.
Izvor: Međunarodna matematička olimpijada, shortlist 2002
Školjka 
from the reals to the reals such that 
for all real 
.