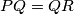
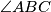
Let be a cyclic quadrilateral. Let , , be the feet of the perpendiculars from to the lines , , , respectively. Show that if and only if the bisectors of and are concurrent with .
%V0
Let $ABCD$ be a cyclic quadrilateral. Let $P$, $Q$, $R$ be the feet of the perpendiculars from $D$ to the lines $BC$, $CA$, $AB$, respectively. Show that $PQ=QR$ if and only if the bisectors of $\angle ABC$ and $\angle ADC$ are concurrent with $AC$.
Izvor: Međunarodna matematička olimpijada, shortlist 2003
Školjka 
be a cyclic quadrilateral. Let 
, 
, 
be the feet of the perpendiculars from 
to the lines 
, 
, 
, respectively. Show that 
if and only if the bisectors of 
and 
are concurrent with 
.