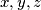
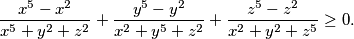
Let be three positive reals such that . Prove that Hojoo Lee, Korea
%V0
Let $x,y,z$ be three positive reals such that $xyz\geq 1$. Prove that
$$\frac { x^5-x^2 }{x^5+y^2+z^2} + \frac {y^5-y^2}{x^2+y^5+z^2} + \frac {z^5-z^2}{x^2+y^2+z^5} \geq 0 .$$
Hojoo Lee, Korea
Izvor: Međunarodna matematička olimpijada, shortlist 2005
Školjka 
be three positive reals such that 
. Prove that 