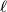
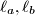
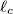
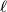
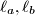
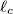
Let be an acute triangle with circumcircle . Let be a tangent line to , and let and be the lines obtained by reflecting in the lines , and , respectively. Show that the circumcircle of the triangle determined by the lines and is tangent to the circle .
Proposed by Japan
%V0
Let $ABC$ be an acute triangle with circumcircle $\Gamma$. Let $\ell$ be a tangent line to $\Gamma$, and let $\ell_a, \ell_b$ and $\ell_c$ be the lines obtained by reflecting $\ell$ in the lines $BC$, $CA$ and $AB$, respectively. Show that the circumcircle of the triangle determined by the lines $\ell_a, \ell_b$ and $\ell_c$ is tangent to the circle $\Gamma$.
Proposed by Japan
Source: Međunarodna matematička olimpijada, shortlist 2011
Školjka 
be an acute triangle with circumcircle 
. Let 
be a tangent line to 
and 
be the lines obtained by reflecting 
, 
and 
, respectively. Show that the circumcircle of the triangle determined by the lines